অয়লার পাথ হল একটি পাথ, যার মাধ্যমে আমরা প্রতিটি প্রান্তে একবার যেতে পারি৷ আমরা একাধিকবার একই শীর্ষবিন্দু ব্যবহার করতে পারি। অয়লার সার্কিট হল একটি বিশেষ ধরনের অয়লার পাথ। যখন অয়লার পথের শুরুর শীর্ষবিন্দুটিও সেই পথের শেষ শীর্ষের সাথে সংযুক্ত থাকে, তখন একে অয়লার সার্কিট বলে।
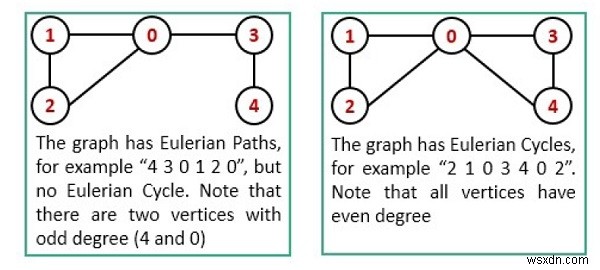
পথ এবং সার্কিট সনাক্ত করতে, আমাদের এই শর্তগুলি অনুসরণ করতে হবে -
- গ্রাফটি অবশ্যই সংযুক্ত থাকতে হবে।
- যখন ঠিক দুটি শীর্ষবিন্দুর বিজোড় ডিগ্রি থাকে, তখন এটি একটি অয়লার পাথ৷
- এখন যখন একটি অনির্দেশিত গ্রাফের কোনো শীর্ষবিন্দুর বিজোড় ডিগ্রি নেই, তখন এটি একটি অয়লার সার্কিট৷
ইনপুট এবং আউটপুট
Input: Adjacency matrix of a graph. 0 1 1 1 0 1 0 1 0 0 1 1 0 0 0 1 0 0 0 1 0 0 0 1 0 Output: The graph has an Eulerian path.
অ্যালগরিদম
ট্রাভার্স(u, পরিদর্শন করা)
ইনপুট: কোন নোডটি পরিদর্শন করা হয়েছে তা চিহ্নিত করতে স্টার্ট নোড u এবং ভিজিট করা নোড।
আউটপুট - সমস্ত সংযুক্ত শীর্ষবিন্দু অতিক্রম করুন৷
৷Begin mark u as visited for all vertex v, if it is adjacent with u, do if v is not visited, then traverse(v, visited) done End
সংযুক্ত (গ্রাফ)
ইনপুট - গ্রাফ।
আউটপুট - গ্রাফ সংযুক্ত থাকলে সত্য।
Begin define visited array for all vertices u in the graph, do make all nodes unvisited traverse(u, visited) if any unvisited node is still remaining, then return false done return true End
isEulerian(গ্রাফ)
ইনপুট - প্রদত্ত গ্রাফ।
আউটপুট - 0 ফেরত দেয়, যখন ইউলারিয়ান নয়, 1 যখন এটির একটি অয়লার পাথ থাকে, 2 যখন অয়লার সার্কিট পাওয়া যায়
Begin if isConnected() is false, then return false define list of degree for each node oddDegree := 0 for all vertex i in the graph, do for all vertex j which are connected with i, do increase degree done if degree of vertex i is odd, then increase dooDegree done if oddDegree > 2, then return 0 if oddDegree = 0, then return 2 else return 1 End
উদাহরণ
#include<iostream>
#include<vector>
#define NODE 5
using namespace std;
int graph[NODE][NODE] = {
{0, 1, 1, 1, 0},
{1, 0, 1, 0, 0},
{1, 1, 0, 0, 0},
{1, 0, 0, 0, 1},
{0, 0, 0, 1, 0}
};
/* int graph[NODE][NODE] = {
{0, 1, 1, 1, 1},
{1, 0, 1, 0, 0},
{1, 1, 0, 0, 0},
{1, 0, 0, 0, 1},
{1, 0, 0, 1, 0}
};
*/ //uncomment to check Euler Circuit
/* int graph[NODE][NODE] = {
{0, 1, 1, 1, 0},
{1, 0, 1, 1, 0},
{1, 1, 0, 0, 0},
{1, 1, 0, 0, 1},
{0, 0, 0, 1, 0}
};
*/ //Uncomment to check Non Eulerian Graph
void traverse(int u, bool visited[]) {
visited[u] = true; //mark v as visited
for(int v = 0; v<NODE; v++) {
if(graph[u][v]) {
if(!visited[v])
traverse(v, visited);
}
}
}
bool isConnected() {
bool *vis = new bool[NODE];
//for all vertex u as start point, check whether all nodes are visible or not
for(int u; u < NODE; u++) {
for(int i = 0; i<NODE; i++)
vis[i] = false; //initialize as no node is visited
traverse(u, vis);
for(int i = 0; i<NODE; i++) {
if(!vis[i]) //if there is a node, not visited by traversal, graph is not connected
return false;
}
}
return true;
}
int isEulerian() {
if(isConnected() == false) //when graph is not connected
return 0;
vector<int> degree(NODE, 0);
int oddDegree = 0;
for(int i = 0; i<NODE; i++) {
for(int j = 0; j<NODE; j++) {
if(graph[i][j])
degree[i]++; //increase degree, when connected edge found
}
if(degree[i] % 2 != 0) //when degree of vertices are odd
oddDegree++; //count odd degree vertices
}
if(oddDegree > 2) //when vertices with odd degree greater than 2
return 0;
return (oddDegree)?1:2; //when oddDegree is 0, it is Euler circuit, and when 2, it is Euler path
}
int main() {
int check;
check = isEulerian();
switch(check) {
case 0: cout << "The graph is not an Eulerian graph.";
break;
case 1: cout << "The graph has an Eulerian path.";
break;
case 2: cout << "The graph has a Eulerian circuit.";
break;
}
} আউটপুট
The graph has an Eulerian path.


